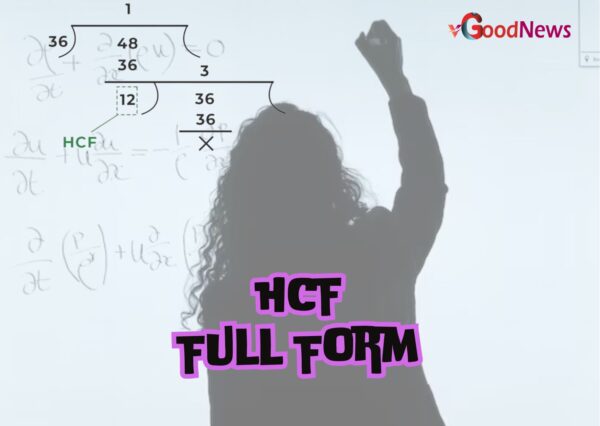
HCF Full Form: The term HCF might seem straightforward, but it’s easy to forget its meaning sometimes. So, let’s clarify that HCF stands for “Highest Common Factor.”
- H = Highest
- C = Common
- F = Factor
HCF = Highest Common Factor
HCF Full Form: What is HCF?
The HCF is a fundamental concept in mathematics, typically introduced around 4th or 5th grade. If you’ve never learned about it or need a refresher, keep reading. I’ll explain how to find the HCF of any number, as well as discuss its full form and the steps involved.
Steps to Find the HCF
Finding the HCF involves identifying the highest number that divides two or more numbers without leaving a remainder. This is a key concept in number theory and helps simplify fractions and solve problems involving divisors. HCF Full Form
Here are the steps to find the HCF of any set of numbers:
- List the Factors: Write down all the factors of each number.
- Identify Common Factors: Look for the common factors in all the lists.
- Select the Highest Factor: Among the common factors, the highest one is the HCF.
By following these steps, you can determine the HCF of any given numbers.
HCF Full Forms – From Different Category
| HCF | Highest Common Factor |
| HCF | Haute Coiffure Francaise |
| HCF | Hardcore Championship Fighting |
| HCF | Highest Common Factor |
| HCF | High Capacity Feeder |
| HCF | Host Controlled Family |
| HCF | Hybrid Cross Fire |
| HCF | Hispanic College Fund |
| HCF | Health Care Facilities |
| HCF | High Cycle Fatigue |
| HCF | Health Care Fraud |
| HCF | Hybrid Coordination Function |
| HCF | Hart Communication Foundation |
| HCF | Hutchinson Correctional Facility |
| HCF | Hamilton Community Foundation |
| HCF | High Capacity Feeder |
| HCF | Hundred Cubic Feet |
| HCF | Historic Charleston Foundation |
| HCF | Home Credit Finance |
| HCF | Hospitals Contribution Fund |
| HCF | Halt and Catch Fire |
| HCF | Health Care Foundation |
| HCF | Hawai’i Community Foundation |
| HCF | Host Controller Free |
| HCF | Harbour City Ferries |
| HCF | Honda Canada Foundation |
| HCF | Host Controlled Family |
| HCF | Half Circle Forward |
| HCF | High-Cost Fund |
| HCF | Highland Credit-Strategies Fund |
| HCF | House Conservatives Fund |
| HCF | Host Command Facility |
| HCF | Heparin Cofactor |
| HCF | Healthy Community Fund |
| HCF | Historic Columbia Foundation |
| HCF | Height Correction Factor |
| HCF | Horizon Christian Fellowship |
| HCF | Hardcore Fan |
| HCF | Hybridoma Cloning Factor |
| HCF | Hepatitis C Foundation |
| HCF | Harper Court Foundation |
| HCF | High Carbon Ferrochrome |
| HCF | Hotline Center Foundation |
| HCF | Hot Channel Factor |
| HCF | Hook Content Formula |
| HCF | Higher Cortical Function |
| HCF | Hardened Compact Fiber |
| HCF | Hardware Configuration Facility |
| HCF | Hard Copy File |
| HCF | Host Computer Facility |
| HCF | Human Care Foundation |
What Is HCF?
HCF stands for Highest Common Factor. It represents the largest factor that two or more numbers have in common. To determine the HCF of any set of numbers, there are several steps involved. HCF Full Form
Before diving into the process of finding the HCF, it’s important to understand a few foundational concepts: What is a factor? How do you find factors? What are prime and composite numbers? What is prime factorization? Understanding these concepts will make the process of finding the HCF much easier.
Key Concepts: HCF Full Form
What is a Factor?
A factor is a number that divides another number without leaving a remainder. For example, 2 and 4 are factors of 8 because 2 × 4 = 8.
Properties of Factors:
- 1 is the smallest factor of any number.
- 1 is the lowest common factor of all numbers.
- The number itself is always the greatest factor.
- All factors of a number are less than or equal to the number.
How to Find Factors
There are two main methods to find factors: Factor Pair Method and Prime Factorization Method.
Factor Pair Method
Multiplication Method:
To find the factors of 24 using multiplication: HCF Full Form
- 1 × 24 = 24
- 2 × 12 = 24
- 3 × 8 = 24
- 4 × 6 = 24
Thus, the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24.
Division Method:
To find the factors of 24 using division: HCF Full Form
- 24 ÷ 1 = 24
- 24 ÷ 2 = 12
- 24 ÷ 3 = 8
- 24 ÷ 4 = 6
- 24 ÷ 6 = 4
- 24 ÷ 8 = 3
- 24 ÷ 12 = 2
- 24 ÷ 24 = 1
Again, the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24.
Prime and Composite Numbers
- Prime Numbers: A prime number is a number greater than 1 that has no factors other than 1 and itself. Examples include 2, 3, 5, 7, and 11.
- Composite Numbers: A composite number is a number greater than 1 that has more than two factors. Examples include 4, 6, 8, 9, and 12.
Prime Factorization
Prime factorization involves breaking down a number into its prime factors. For instance, the prime factorization of 24 is: 24 = 2 × 2 × 2 × 3 = 2^3 × 3
There are two types of Method
- Factor Tree Method of Prime Factorisation.
- Common Division Method of Prime Factorisation.
Finding Common Factors and HCF
To find the HCF of two or more numbers, you need to identify the common factors among them and select the highest one.
Steps to Find the HCF
- List the Factors: Identify all the factors of each number.
- Find Common Factors: Determine which factors are common to all the numbers.
- Select the Highest Common Factor: The largest common factor is the HCF.
Finding the prime factorization of a number can be straightforward if you use the right methods. Here, we’ll discuss two common techniques: the Factor Tree method and the Common Division method. HCF Full Form
Prime Factorization Using a Factor Tree
The Factor Tree method is a simple and visual way to break down a number into its prime factors. Let’s walk through the steps using the number 24 as an example. HCF Full Form
- Start with the smallest prime factor of 24: 2.
- Divide 24 by 2: This gives us 12.
- Find the smallest prime factor of 12: 2.
- Divide 12 by 2: This gives us 6.
- Find the smallest prime factor of 6: 2.
- Divide 6 by 2: This gives us 3.
- 3 is a prime number: We stop here.
So, the prime factorization of 24 is: 24=2×2×2×3=23×3
The Factor Tree method helps to visually see the step-by-step breakdown of the number into its prime components.
Prime Factorization Using Common Division
The Common Division method is another straightforward approach to prime factorization, focusing on division. HCF Full Form
- Start by dividing 24 by the smallest prime factor, 2: This gives us 12.
- Divide 12 by 2: This gives us 6.
- Divide 6 by 2: This gives us 3.
- 3 is a prime number: We stop here.
So, the prime factorization of 24 using common division is also: 24=2×2×2×3=23×3
This method emphasizes repeated division by the smallest prime numbers until you reach a prime number.
Finding Common Factors
To find common factors, you need to compare the factors of two or more numbers. Let’s use the numbers 24 and 32 as an example. HCF Full Form
List the factors of each number:
- Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
- Factors of 32: 1, 2, 4, 8, 16, 32
Identify the common factors:
- Common factors of 24 and 32: 1, 2, 4, 8
Finding the Highest Common Factor (HCF)
To find the HCF, you identify the highest number in the list of common factors. Let’s find the HCF of 32 and 44.
List the factors of each number:
- Factors of 32: 1, 2, 4, 8, 16, 32
- Factors of 44: 1, 2, 4, 11, 22, 44
Identify the common factors:
- Common factors of 32 and 44: 1, 2, 4
Select the highest common factor:
- HCF: 4
Thus, the highest common factor (HCF) of 32 and 44 is 4.
Conclusion
Understanding the HCF (Highest Common Factor) is essential for solving various mathematical problems. By learning to identify factors, perform prime factorization, and find common factors, you can easily determine the HCF (HCF Full Form) of any set of numbers. This fundamental concept is not only crucial in mathematics but also in simplifying fractions and solving problems related to divisors.